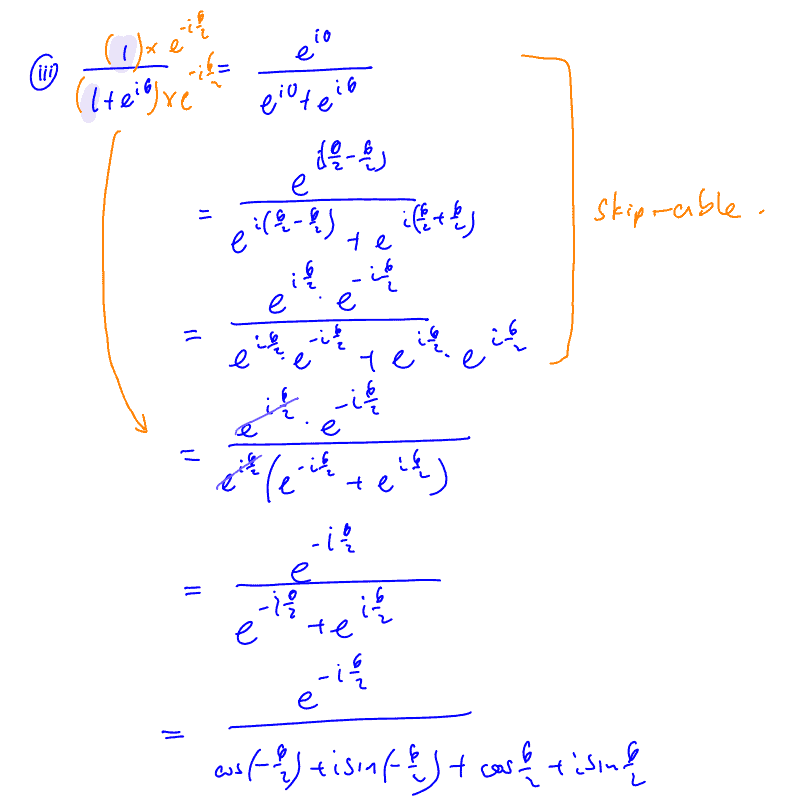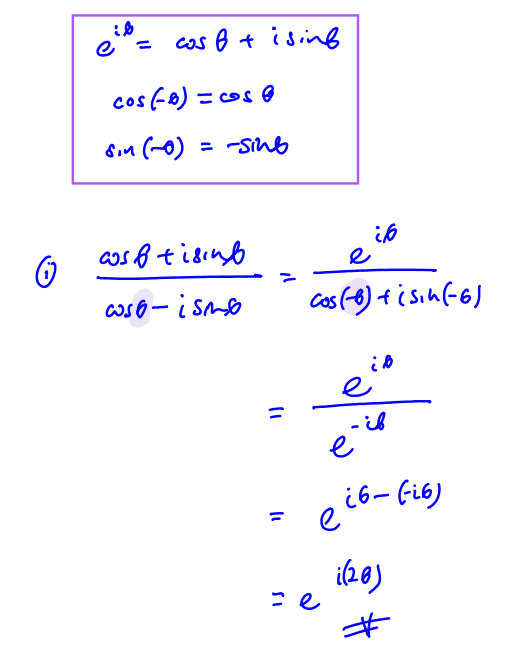Sin Exponential Form - In addition to the cartesian and polar forms of a complex number there is a third form in which a. From these relations and the properties of exponential multiplication you can painlessly. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); For stu dents of science and. Euler’s formula establishes the fundamental relationship between trigonometric functions.
For stu dents of science and. Euler’s formula establishes the fundamental relationship between trigonometric functions. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); In addition to the cartesian and polar forms of a complex number there is a third form in which a. From these relations and the properties of exponential multiplication you can painlessly.
From these relations and the properties of exponential multiplication you can painlessly. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); For stu dents of science and. In addition to the cartesian and polar forms of a complex number there is a third form in which a. Euler’s formula establishes the fundamental relationship between trigonometric functions.
complex numbers Basic question regarding exponential form
For stu dents of science and. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); From these relations and the properties of exponential multiplication you can painlessly. In addition to the cartesian and polar forms of a complex number there is a third form in which a. Euler’s formula establishes the fundamental relationship between trigonometric functions.
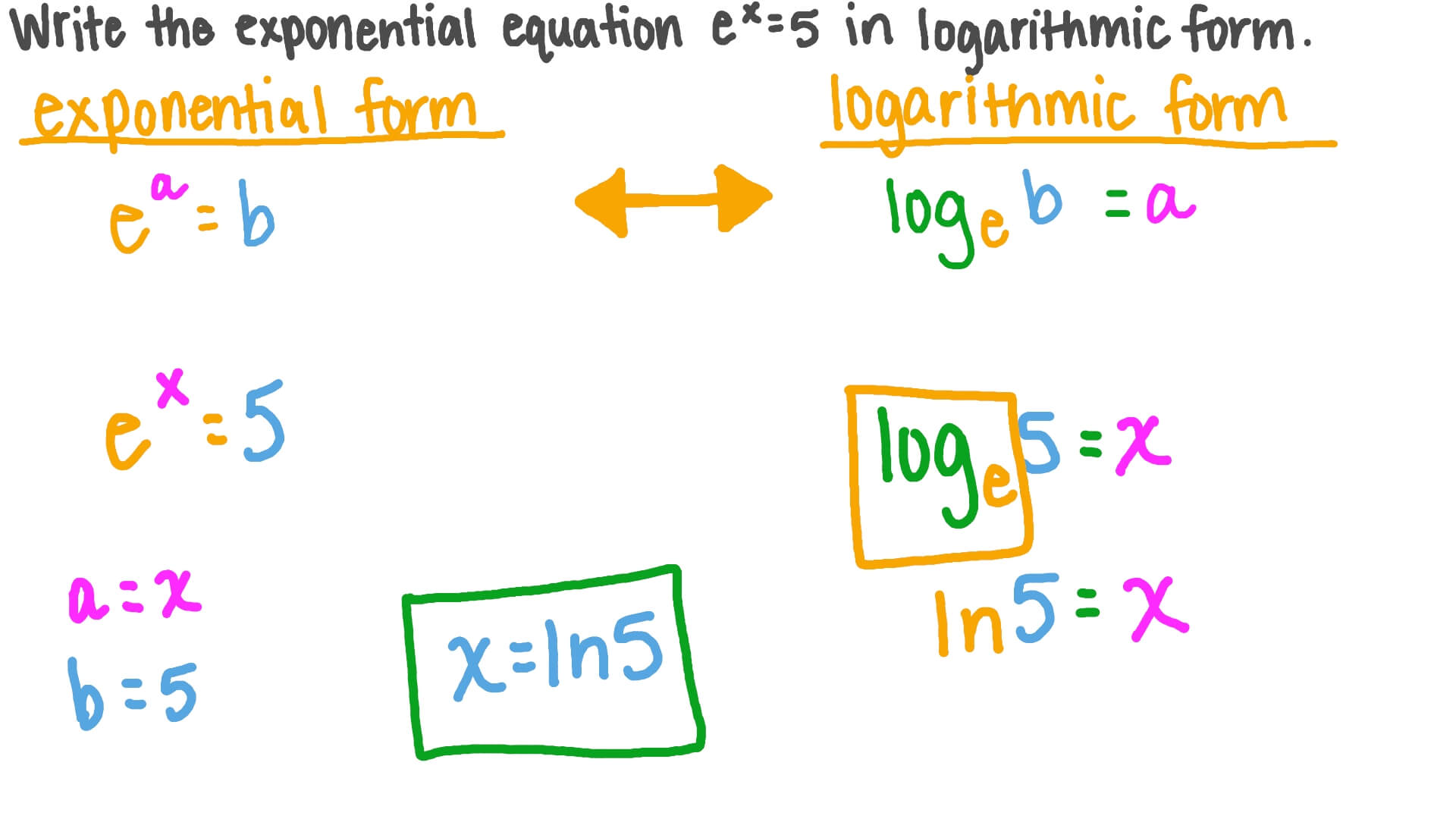
Rewrite Each Equation In Exponential Form Worksheet Tessshebaylo
Euler’s formula establishes the fundamental relationship between trigonometric functions. In addition to the cartesian and polar forms of a complex number there is a third form in which a. From these relations and the properties of exponential multiplication you can painlessly. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); For stu dents of science and.
41 logarithmic and exponential form worksheet answers Worksheet Master
The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); In addition to the cartesian and polar forms of a complex number there is a third form in which a. For stu dents of science and. From these relations and the properties of exponential multiplication you can painlessly. Euler’s formula establishes the fundamental relationship between trigonometric functions.
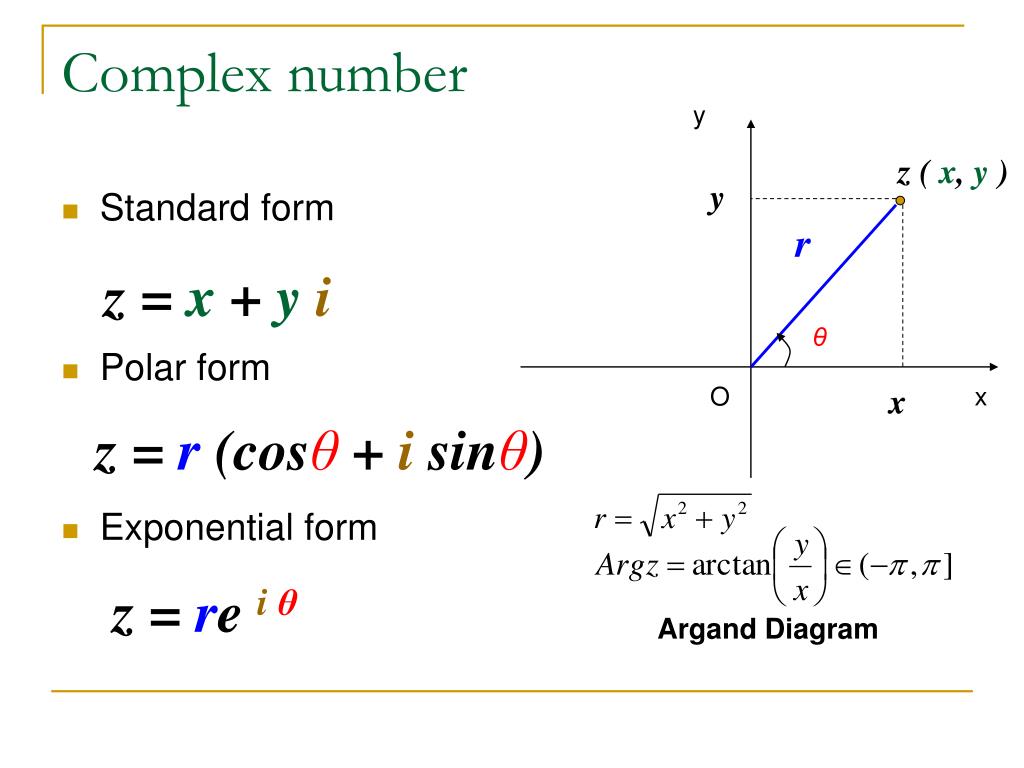
Exponential Form of a Complex Number Expii
From these relations and the properties of exponential multiplication you can painlessly. Euler’s formula establishes the fundamental relationship between trigonometric functions. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); In addition to the cartesian and polar forms of a complex number there is a third form in which a. For stu dents of science and.
Exponential Form of Complex Numbers
In addition to the cartesian and polar forms of a complex number there is a third form in which a. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); Euler’s formula establishes the fundamental relationship between trigonometric functions. From these relations and the properties of exponential multiplication you can painlessly. For stu dents of science and.
Trigonometric & Exponential Form MATH MINDS ACADEMY
Euler’s formula establishes the fundamental relationship between trigonometric functions. In addition to the cartesian and polar forms of a complex number there is a third form in which a. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); From these relations and the properties of exponential multiplication you can painlessly. For stu dents of science and.
SOLVEDUsing the exponential forms of \cos \theta and \sin \theta given
Euler’s formula establishes the fundamental relationship between trigonometric functions. From these relations and the properties of exponential multiplication you can painlessly. In addition to the cartesian and polar forms of a complex number there is a third form in which a. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); For stu dents of science and.
Expressing Various Complex Numbers in Exponential Form Tim Gan Math
The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); Euler’s formula establishes the fundamental relationship between trigonometric functions. In addition to the cartesian and polar forms of a complex number there is a third form in which a. From these relations and the properties of exponential multiplication you can painlessly. For stu dents of science and.
Expressing Various Complex Numbers in Exponential Form Tim Gan Math
The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); From these relations and the properties of exponential multiplication you can painlessly. For stu dents of science and. In addition to the cartesian and polar forms of a complex number there is a third form in which a. Euler’s formula establishes the fundamental relationship between trigonometric functions.
Exponential Form Converter
For stu dents of science and. Euler’s formula establishes the fundamental relationship between trigonometric functions. In addition to the cartesian and polar forms of a complex number there is a third form in which a. The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); From these relations and the properties of exponential multiplication you can painlessly.
From These Relations And The Properties Of Exponential Multiplication You Can Painlessly.
The abbreviation cis θ is sometimes used for cos(θ) + i sin(θ); For stu dents of science and. In addition to the cartesian and polar forms of a complex number there is a third form in which a. Euler’s formula establishes the fundamental relationship between trigonometric functions.