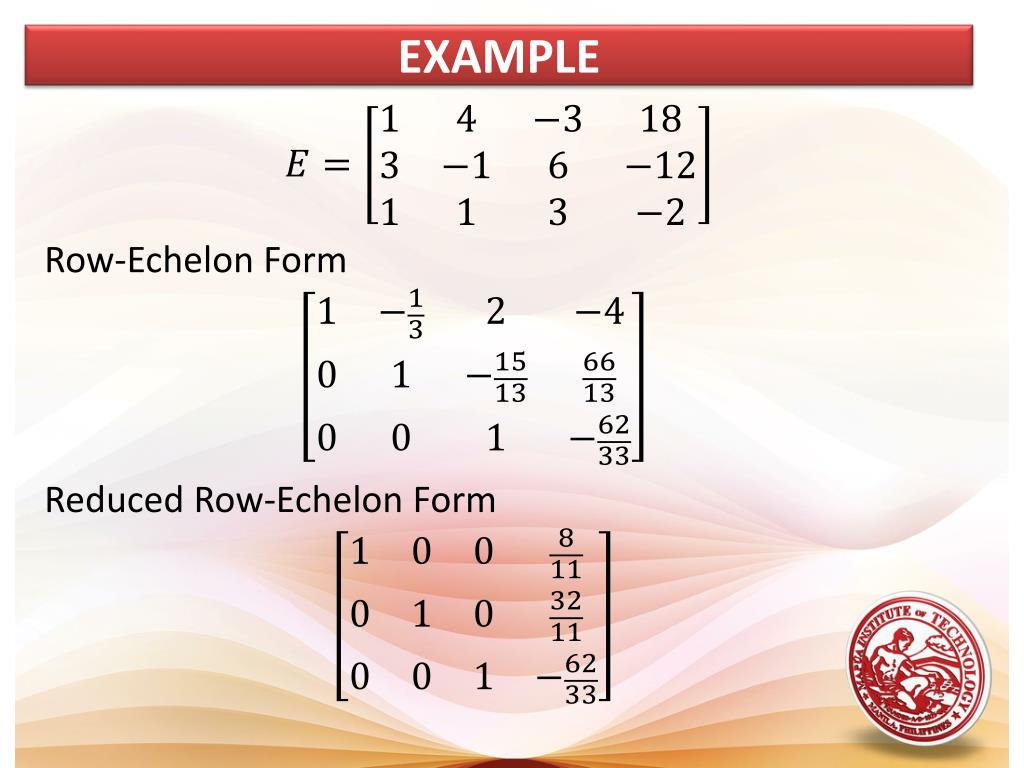
Symbolab Echelon Form - The gauss jordan elimination is a method of putting a matrix in row reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row. In the last two blog posts, we talked about row echelon form (ref) and reduced. The gaussian elimination, is a method of putting a matrix in row echelon form.
In the last two blog posts, we talked about row echelon form (ref) and reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row. The gauss jordan elimination is a method of putting a matrix in row reduced. The gaussian elimination, is a method of putting a matrix in row echelon form.
The gaussian elimination, is a method of putting a matrix in row echelon form. We use row operations corresponding to equation operations to obtain a new matrix that is row. The gauss jordan elimination is a method of putting a matrix in row reduced. In the last two blog posts, we talked about row echelon form (ref) and reduced.
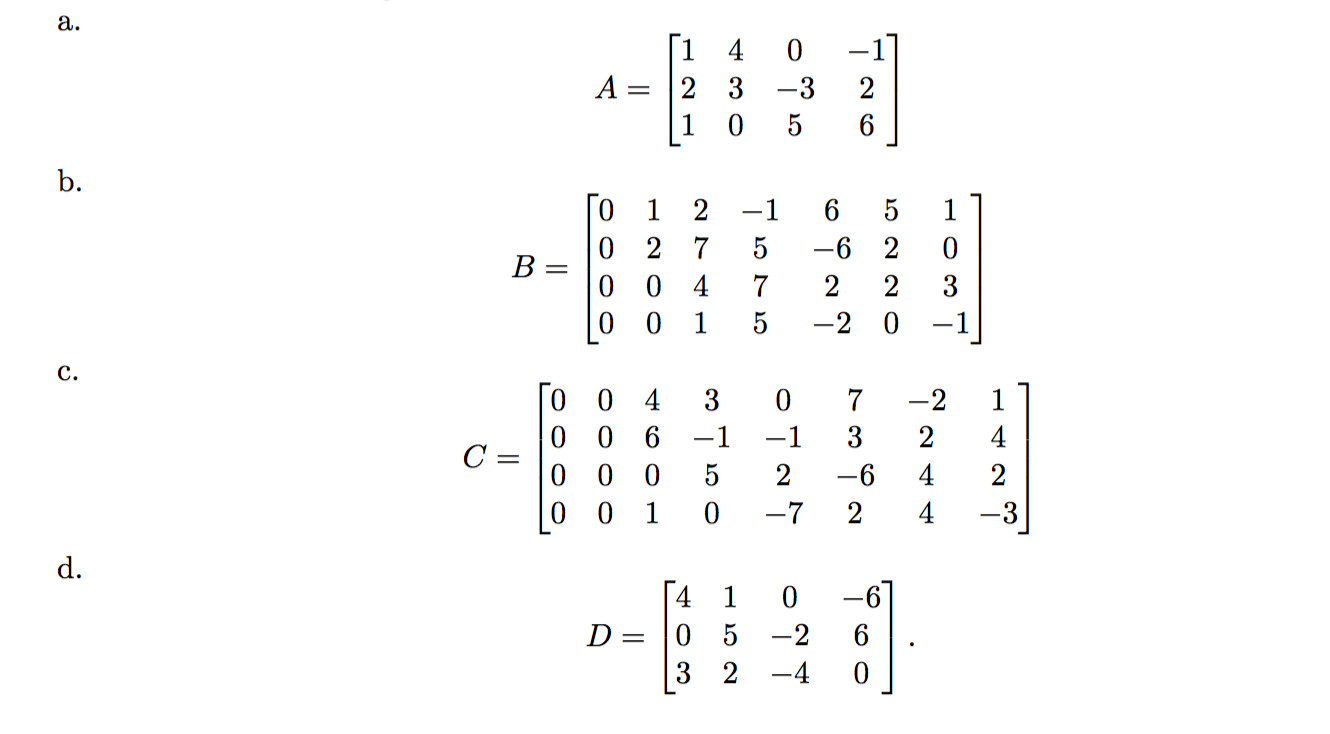
Row Echelon Form Vs Reduced Row Echelon Form, 57 OFF
The gauss jordan elimination is a method of putting a matrix in row reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row. The gaussian elimination, is a method of putting a matrix in row echelon form. In the last two blog posts, we talked about row echelon form (ref) and reduced.
Echelon Form
We use row operations corresponding to equation operations to obtain a new matrix that is row. In the last two blog posts, we talked about row echelon form (ref) and reduced. The gauss jordan elimination is a method of putting a matrix in row reduced. The gaussian elimination, is a method of putting a matrix in row echelon form.
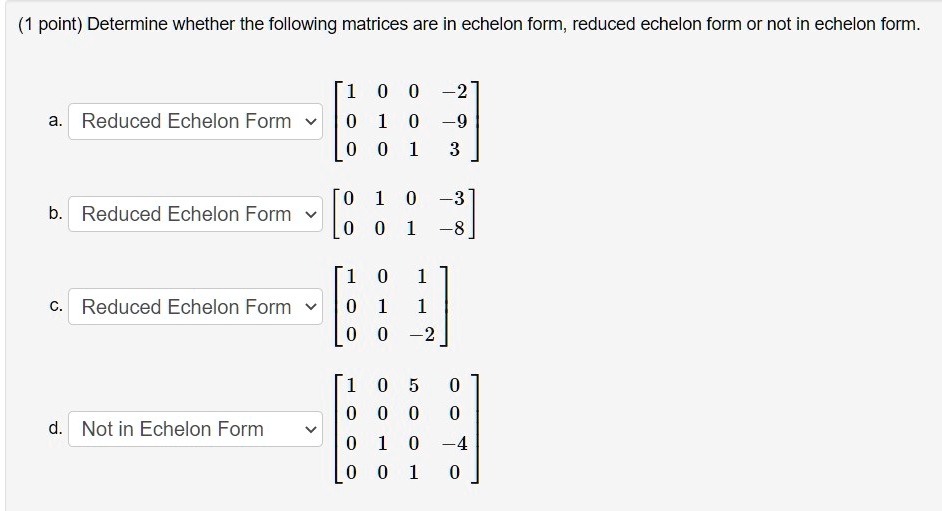
Solved Determine whether the following matrices are in
The gauss jordan elimination is a method of putting a matrix in row reduced. In the last two blog posts, we talked about row echelon form (ref) and reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row. The gaussian elimination, is a method of putting a matrix in row echelon form.
Echelon Form
The gauss jordan elimination is a method of putting a matrix in row reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row. In the last two blog posts, we talked about row echelon form (ref) and reduced. The gaussian elimination, is a method of putting a matrix in row echelon form.
Echelon Form Calculator
The gaussian elimination, is a method of putting a matrix in row echelon form. We use row operations corresponding to equation operations to obtain a new matrix that is row. The gauss jordan elimination is a method of putting a matrix in row reduced. In the last two blog posts, we talked about row echelon form (ref) and reduced.
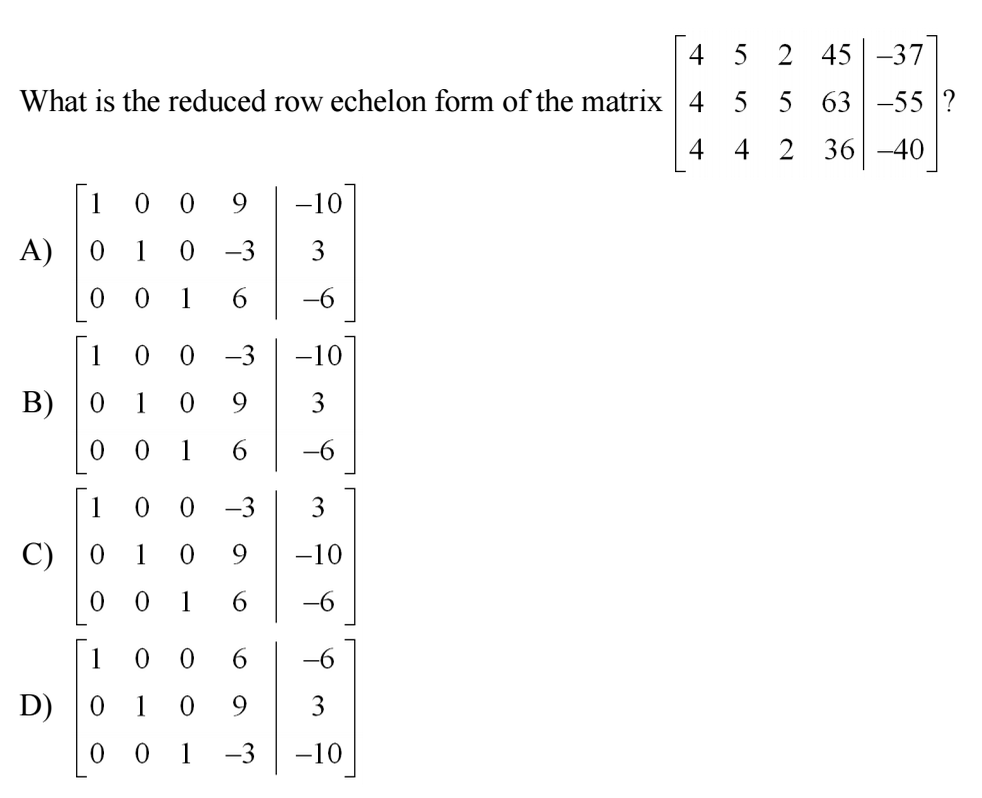
SOLVED point) Determine whether the following matrices are in echelon
The gauss jordan elimination is a method of putting a matrix in row reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row. The gaussian elimination, is a method of putting a matrix in row echelon form. In the last two blog posts, we talked about row echelon form (ref) and reduced.
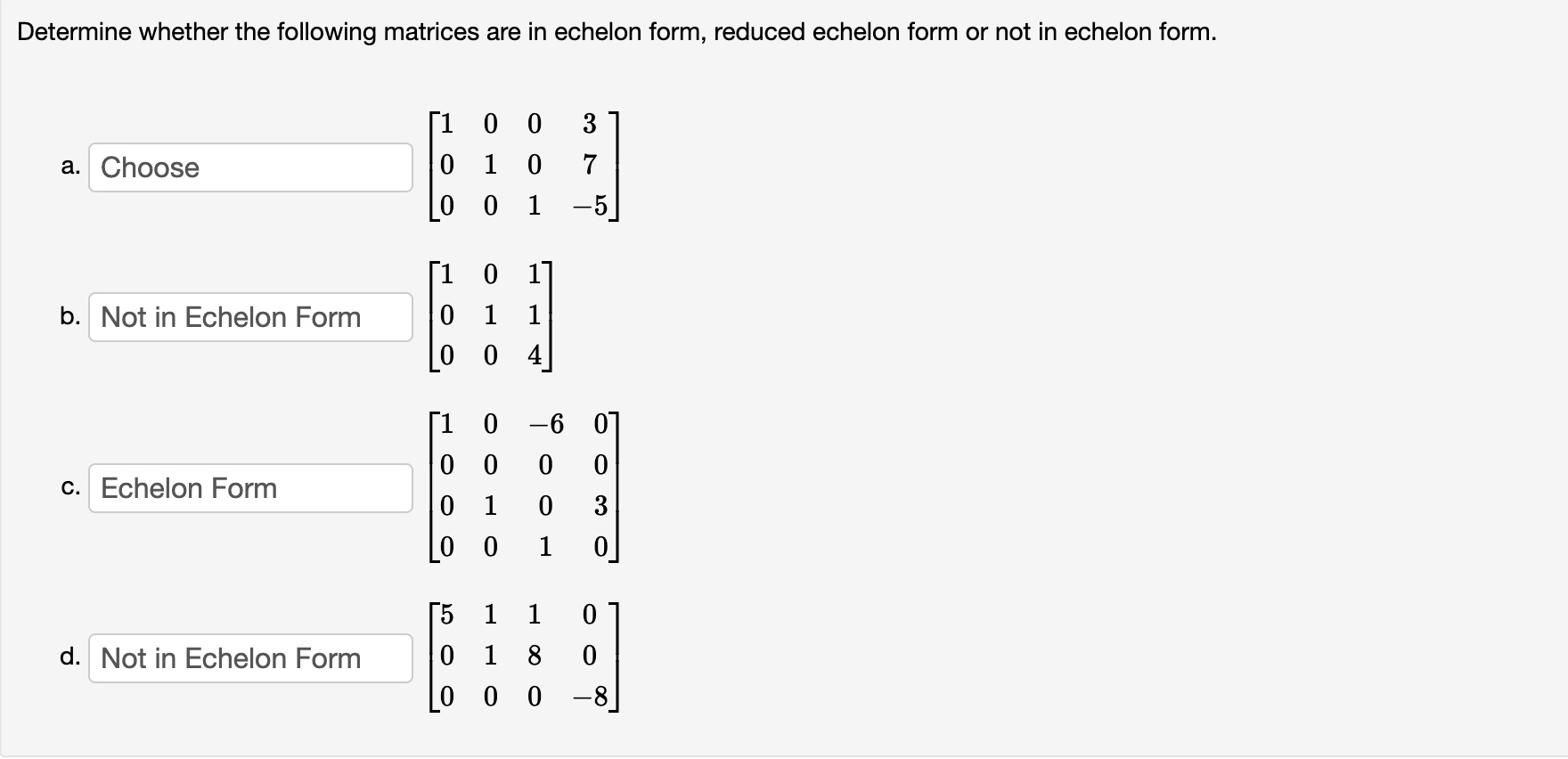
Row and Reduced Echelon Form PDF Matrix (Mathematics) Theoretical
The gauss jordan elimination is a method of putting a matrix in row reduced. The gaussian elimination, is a method of putting a matrix in row echelon form. In the last two blog posts, we talked about row echelon form (ref) and reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row.
Row Echelon Form Vs Reduced Row Echelon Form, 57 OFF
We use row operations corresponding to equation operations to obtain a new matrix that is row. In the last two blog posts, we talked about row echelon form (ref) and reduced. The gauss jordan elimination is a method of putting a matrix in row reduced. The gaussian elimination, is a method of putting a matrix in row echelon form.
Solved Determine whether the following matrices are in
We use row operations corresponding to equation operations to obtain a new matrix that is row. The gaussian elimination, is a method of putting a matrix in row echelon form. In the last two blog posts, we talked about row echelon form (ref) and reduced. The gauss jordan elimination is a method of putting a matrix in row reduced.
Echelon Form
The gauss jordan elimination is a method of putting a matrix in row reduced. The gaussian elimination, is a method of putting a matrix in row echelon form. In the last two blog posts, we talked about row echelon form (ref) and reduced. We use row operations corresponding to equation operations to obtain a new matrix that is row.
The Gauss Jordan Elimination Is A Method Of Putting A Matrix In Row Reduced.
In the last two blog posts, we talked about row echelon form (ref) and reduced. The gaussian elimination, is a method of putting a matrix in row echelon form. We use row operations corresponding to equation operations to obtain a new matrix that is row.